MVPstats — Proportion Tests Two-Sample

The Two-sample Proportion tests are used to test if the samples were drawn from a populations with equal proportions. Both exact and approximate tests may be performed.

Hypotheses

The following hypotheses may be tested:

Where and are the population proportions from which samples were drawn.

Assumptions

1. The samples has been randomly drawn from two independent populations (Critical)
2. The populations may be modeled with a binomial distribution, which has these attributes:
   1. Only two possible outcomes or classifications occur with each item examined (Critical)
   2. The probability of occurrence of each classification remains fixed from item to item over time (Critical)
   3. The probability of occurrence of each item sampled is independent of all other items sampled (Critical)

Test Statistics

Approximate Test

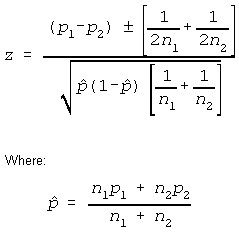
Where the +/- sign is determined as follows for the above hypothesis:

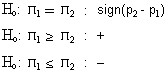
Fisher Exact Test

If we let:

Assuming a+b, c+d, a+c, and b+d as fixed, then the probability of obtaining exactly a and b within the sample is:

This represents the probability of exactly a and b occurrences. These probabilities are calculated for all possible results departing as much or more as a and b. The sum of these probabilities is the one-tailed significance. For two-sided tests the probabilities are calculated for all possible cases where the following is as great or greater than that observed:

Output

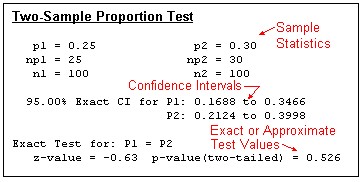
Note

The p-value is flagged with an asterisk (*) when p <= alpha.