The Correlation Test for Rho = 
is used to test if a correlation exists between two variables of some specific value. The correlation represents the degree to which a linear relationship exists between these two variables. If the desired test is for a equal to zero, see the Test for Correlation = 0.
Hypotheses
The following hypotheses may be tested:
Where 
is the true population correlation from which the sample was drawn, and is some value other than zero between -1.00 and 1.00.
Assumptions
1. The sample has been randomly drawn from a bivariate (two-dimensional) population (Critical)
2. The population may be modeled with a bivariate normal distribution (Not Critical, if unimodal)
3. The correlation tested represents the degree to which a linear relationship exists between the two variables (Critical)
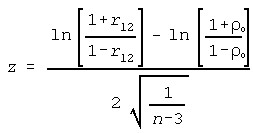
Test Statistics
Output
Note
The p-value is flagged with an asterisk (*) when p <= alpha.